SOCR EduMaterials Activities Binomial PGF
From Socr
(Difference between revisions)
(New page: == This is an activity to explore the Probability Generating Functions for the Bernoulli, Binomial, Geometric and Negative-Binomial Distributions.== * '''Description''': You can access t...) |
|||
| Line 10: | Line 10: | ||
Below you can see a snapshot of the PGF of the distribution of <math> X \sim Bernoulli(0.8) </math> | Below you can see a snapshot of the PGF of the distribution of <math> X \sim Bernoulli(0.8) </math> | ||
| - | <center>[[Image: | + | <center>[[Image:BernoulliPGF1.jpg|600px]]</center> |
Do you notice any similarities between the graphs of these PGF's between any of these distributions? | Do you notice any similarities between the graphs of these PGF's between any of these distributions? | ||
| Line 17: | Line 17: | ||
* '''Exercise 3:''' You learned in class about the properties of PGF's If <math> X_1, ...X_n</math> are iid. and <math>Y = \sum_{i=1}^n X_i. </math> then <math>P_{y}(t) = {[P_{X_1}(t)]}^n</math>. | * '''Exercise 3:''' You learned in class about the properties of PGF's If <math> X_1, ...X_n</math> are iid. and <math>Y = \sum_{i=1}^n X_i. </math> then <math>P_{y}(t) = {[P_{X_1}(t)]}^n</math>. | ||
| - | **a. Show that the PGF of the sum of <math>n</math> independent Bernoulli Trials with success probability <math> p </math> is the same as the | + | **a. Show that the PGF of the sum of <math>n</math> independent Bernoulli Trials with success probability <math> p </math> is the same as the PGF of the Binomial Distribution using the corollary above. |
**b. Show that the PGF of the sum of <math>n</math> independent Geometric Random Variables with success probability <math> p </math> is the same as the MGF of the Negative-Binomial Distribution using the corollary above. | **b. Show that the PGF of the sum of <math>n</math> independent Geometric Random Variables with success probability <math> p </math> is the same as the MGF of the Negative-Binomial Distribution using the corollary above. | ||
| - | **c. How does this relate to Exercise 1? Does having the same | + | **c. How does this relate to Exercise 1? Does having the same PGF mean they are distributed the same? |
Revision as of 03:33, 9 January 2008
This is an activity to explore the Probability Generating Functions for the Bernoulli, Binomial, Geometric and Negative-Binomial Distributions.
- Description: You can access the applets for the above distributions at http://www.socr.ucla.edu/htmls/SOCR_DistributionFunctors.html .
- Exercise 1: Use SOCR to graph the PGF's and print the following distributions and answer the questions below. Also, comment on the shape of each one of these distributions:
- a.

- b.
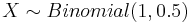
- c.

- d.
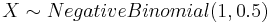
- a.
Below you can see a snapshot of the PGF of the distribution of 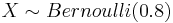
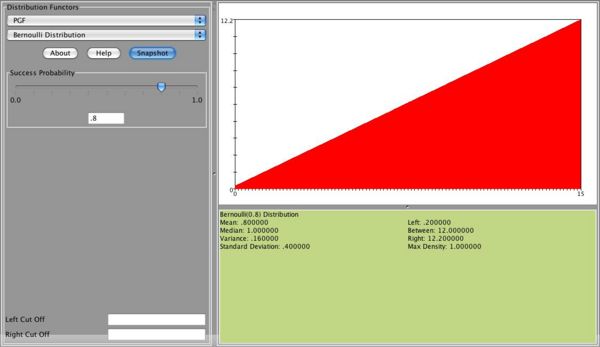
Do you notice any similarities between the graphs of these PGF's between any of these distributions?
- Exercise 2: Use SOCR to graph and print the PGF of the distribution of a geometric random variable with p = 0.2,p = 0.7. What is the shape of this function? What happens when p is large? What happens when p is small?
- Exercise 3: You learned in class about the properties of PGF's If X1,...Xn are iid. and
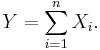 then
then ![P_{y}(t) = {[P_{X_1}(t)]}^n](/socr/uploads/math/4/4/7/44715cd09714f0e09436cdd22aa5b660.png) .
.
- a. Show that the PGF of the sum of n independent Bernoulli Trials with success probability p is the same as the PGF of the Binomial Distribution using the corollary above.
- b. Show that the PGF of the sum of n independent Geometric Random Variables with success probability p is the same as the MGF of the Negative-Binomial Distribution using the corollary above.
- c. How does this relate to Exercise 1? Does having the same PGF mean they are distributed the same?
